UnitMath allows you to easily do calculations and get results you can trust.
Examples:
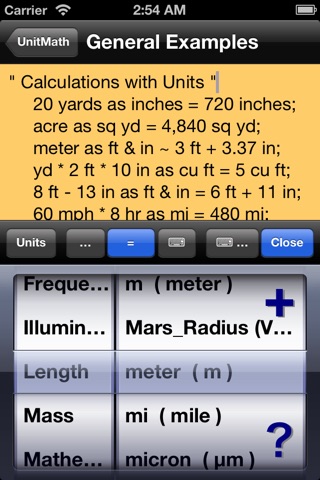
1 + 2 + 3 + 4 = 10;
60 mph * 2 minutes as yards = 14,080 yards;
40,500 km / ( 100 km / hr ) as days & hr = 16 days + 21 hr;
1/4 inch * acre as cu yd ~ 33.6 cu yd;
UnitMath is a giant leap beyond disjoint unit conversions and calculations to a world of seamless calculations with units. Enter your equations with the units you know and ask for the results in the units you want.
UnitMath is a giant leap beyond disjoint unit conversions and calculations to a world of seamless calculations with units. Enter your equations with the units you know and ask for the results in the units you want.
Usefulness
UnitMath is useful for a wide range of people from the casual layman to world class scientists and engineers. Whoever you are, and whatever your level, you know concepts and formulas that enable you to use UnitMath as a powerful tool – the more you know the more useful you will find UnitMath. UnitMath’s intuitive interface and extensive documentation make it easy to use.
With UnitMath you use what you know to do calculations that would be too messy to do otherwise, and you will have real confidence in your answers. The confidence comes from
•Seeing the entire equation, so you know it was entered correctly
•Entering data in the units you measure eliminating conversion errors
•Easily testing your equations against known data
•Seeing results in units you are familiar with, so you can spot errors of kind and quantity.
In UnitMath errors of kind are obvious: if your answer comes out in meters when you expected seconds you know you have a problem. Errors of quantity are harder, but when the result is in units you are familiar with, your common sense often helps ( what is the volume of an average person – less than a cubic foot is too small – more than 10 cubic feet is too large ).
Units
UnitMath knows hundreds of constants (meters, grams, seconds, etc. ). Each constant may use any standard prefix (megaMeters, kilograms, nanoSeconds, etc.), and constants can be combined by operators to form compound units (m/s, sq m, kg * m / hr, etc. ). UnitMath also allows user-defined variables which act much like constants or functions. Variables make equations faster to enter, less prone to errors, and simpler to understand. With constants, operators and variables the number of units UnitMath can use is unlimited.
Numbers
UnitMath knows and can calculate with integers, reals, exponentials, complex numbers, phasor notation, & vectors.
Examples:
e^( i pi ) = -1;
20 V phase(90°) * 2 A phase(45°) = 40 phase ( 135 ° ) W;
( 2 ft x̂ cross ( 150 lb gn ŷ ) as N m; ~ 406.7 ẑ N m;
Uncertainty
UnitMath also helps you – “Know What You Know” – ‘4.0853334 hours’ is interesting, ‘4 hr + 5 min + 7.2 s’ is more meaningful but ‘( 3.5 to 4.7 ) hours’ is much more useful. With UnitMath you can easily find the uncertainty (the give or take) in your answer. When you know the uncertainty of your answer you “Know what you know”.
Example:
distance is ( 230 to 260 ) mi;
speed is ( 55 to 65 ) mph;
distance / speed as hr ~ ( 3.5 to 4.7 ) hr;
Conclusion
In UnitMath use what you know: measured data, concepts & formulas – Get the information you need: in the units you will use & knowing the range of the result. UnitMath enables you to easily use the concepts you know to do useful calculations.
I hope you find UnitMath useful and fun.